> Example of transformation array that appeared 6 times
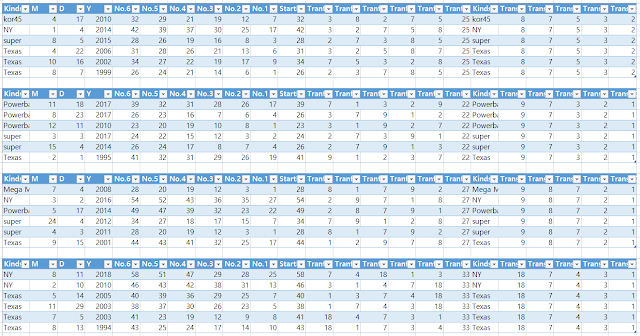
The sum of the transforms is 25, so the array is 8 7 5 3 2
The sum of the transformations is 22 and the array is 9 7 3 2 1
The sum of the transformations is 27 and the array is 9 8 7 2 1
The sum of the transformations is 33, and the array is 18 7 4 3 1
For example, if you continued to apply after August 2011 from the starting point 26 to 43 with a sequence of 22 9 7 3 2 1, you won in November 2017. Of course, it’s a belated subjunctive
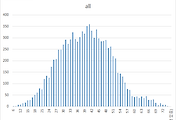
We already know that the sum of the transforms represents the normal distribution that draws the vertices at 41.
In other words, when the sum of the transforms is greater than 41, the size of the transform value increases and the variable at the start point becomes small, but if the sum of the transforms is less than 41, the size of the transform decreases but the variable at the start point increases.
Now, then, let's consider with the fact that in the case of Korea45, the sum of the current transformations shows a relatively very low appearance at 35, 36, and 39.
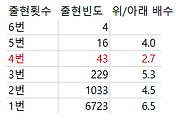
You just need to fit 5 sides, that is, 5 transform values.
The starting point is 26-45 or 30-45, or 35-45, or 40-45 to take less.
Now we have to select the transform value, i.e. the values of the five sides, first of all, recall that we have summed the transforms.
This is to find the case where the sum of the transformations is 35, 36, 39.
In this case, when the sum of the transformations is 35, if the starting point is set as the number 41, the remaining number, that is, the smallest number is determined. This is the same reason as the starting point and the ending point are determined because the agreement of the sides is decided.
If so, you can now set the number that fits the position of the conversion values 1, 2, 3, 4.
In other words, you just need to set the five most probable transforms where the sum of the transforms is 35.
In the case of the Texas lotto above, in the case of the pattern 18 7 4 3 1 with a sum of 33 transforms, the transformed value 18 4 7 3 1 with a starting point of 41 was won.
Let's decide what to do as we continue analyzing the pattern.
> 6번 출현했던 변환 배열의 예
변환의 합이 25로 배열이 8 7 5 3 2
변환의 합이 22로 배열이 9 7 3 2 1
변환의 합이 27로 배열이 9 8 7 2 1
변환의 합이 33로 배열이 18 7 4 3 1
일례로, 변환의 합이 22인 배열 9 7 3 2 1로 시작점 26~43까지 2011년 8월이후 계속 응모했다면 2017년 11월에 당첨되었다. 물론 뒤늦은 가정법이지만
변환의 합이 41에서 정점을 그리는 정규분포를 나타냄을 우리는 이미 알고 있다.
다시말해, 변환의 합이 41이상될 경우 변환의 값 크기가 크게 되어 시작점의 변수는 작아지지만, 변환의 합이 41보다 작은 경우에는 변환의 크기는 작아지지만 시작점의 변수가 커진다.
자, 그렇다면 korea45의 경우 현재 변환의 합이 35, 36, 39에서 상대적으로 매우 낮은 출현을 나타내고 있다는 사실을 갖고서 고민해보자
5개의 변, 즉 변환값 5개를 맞추면 된다.
시작점은 26~45 이거나 30~45, 또는 35~45, 더 적게 가져가려면 40~45를 선택해도 좋다.
이제 변환값, 즉 5개의 변 값을 선택해야 하는데 우선, 우리는 변환의 합을 정했다는 점을 먼저 상기하자
변환의 합이 35, 36, 39인 경우를 찾는 것이다.
이 경우 변환의 합이 35인 때 시작점을 숫자 41로 정하면 나머지 한 숫자, 즉 가장 작은 숫자가 정해진다. 왜냐하면 변의합을 정했기 때문에 시작점과 끝 점이 정해지는 것과 같은 이치다.
그렇다면, 이제 변환 값 1, 2, 3, 4의 위치에 맞는 수를 정하면 된다.
다시말해, 변환의 합이 35를 나타내는 가장 그럴 듯한 5개의 변환값을 정하면 된다.
위의 Texas lotto의 경우 변환의합이 33인 패턴 18 7 4 3 1의 경우 시작점을 41로 하는 변환값 18 4 7 3 1로 당첨되었다.
구체적인 건 계속 패턴을 분석해 나가면서 정하기로 하자.
'현금흐름 > Lotto의 실마리, 2011.8.~' 카테고리의 다른 글
| Trend when sorting in descending order of converted value (0) | 2021.01.14 |
|---|---|
| Korea 45 ball trend to see (0) | 2021.01.14 |
| Trend of appearance in descending order of transform array (0) | 2021.01.14 |
| Conversion value trend (0) | 2021.01.14 |
| Recent powerball trends (~2020.12.19.) (0) | 2021.01.14 |

댓글